Sometimes, the fourth dimension is interpreted in the spatial sense: a space with literally 4 spatial dimensions, 4 mutually orthogonal directions of movement. This is the space used by mathematicians when studying geometric objects such as 4-dimensional polytopes. To avoid confusion with the more common Einsteinian notion of time being the fourth dimension, however, the use of this spatial interpretation should be stated at the outset.
Mathematically, the 4-dimensional spatial equivalent of conventional 3-dimensional geometry is the Euclidean 4-space, a 4-dimensional normed vector space with the Euclidean norm. The "length" of a vector
expressed in the standard basis is given by
which is the natural generalization of the Pythagorean Theorem to 4 dimensions. This allows for the definition of the angle between two vectors (see Euclidean space for more information).
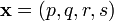

No comments:
Post a Comment